Fields Medals 2022
The Fields Medal is awarded to recognize outstanding mathematical achievement for existing work and for the promise of future achievement.
The medals and cash prizes are funded by a trust established by J.C.Fields at the University of Toronto, which has been replenished periodically, but is still significantly underfunded. In 2022, the prize funds from the University of Toronto were supplemented by generous support from the Heidelberg Laureate Forum Foundation/Klaus Tschira Stiftung.
Hugo Duminil-Copin
For solving longstanding problems in the probabilistic theory of phase transitions in statistical physics, especially in dimensions three and four.
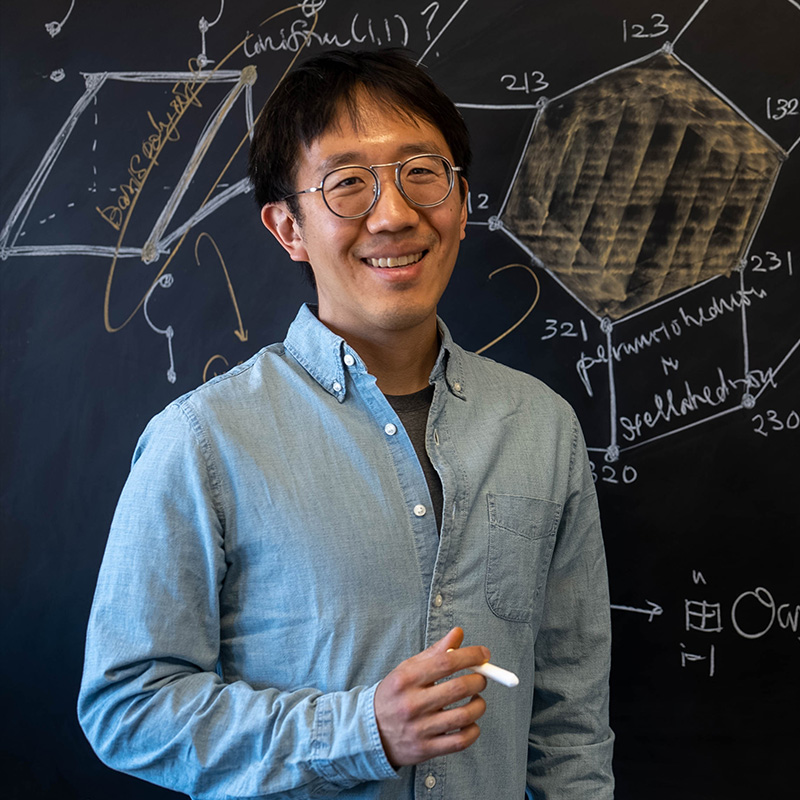
June Huh
For bringing the ideas of Hodge theory to combinatorics, the proof of the Dowling–Wilson conjecture for geometric lattices, the proof of the Heron–Rota–Welsh conjecture for matroids, the development of the theory of Lorentzian polynomials, and the proof of the strong Mason conjecture.
James Maynard
For contributions to analytic number theory, which have led to major advances in the understanding of the structure of prime numbers and in Diophantine approximation.
Maryna Viazovska
For the proof that the E8 lattice provides the densest packing of identical spheres in 8 dimensions, and further contributions to related extremal problems and interpolation problems in Fourier analysis.