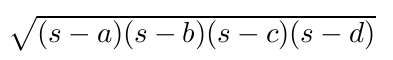Indian Bid to Host the ICM 2010
Presentation by the Indian Delegation
to
the General Assembly of the IMU, 2006
Santiago de Compostela, Spain
August 20, 2006
S. G. Dani, R. Hans-Gill, S. Kesavan and G. Misra
Large community of working mathematicians. Many leaders
in their
fields of research.
Several have been closely associated with the activities of the IMU.
Long tradition of mathematical research, spanning over three
millenia.
State of the art facilities available for holding large meetings.
Marvelous tourist destination.
Ancient Indian Mathematics
Earliest explicit statement of what is called 'Pythagoras' theorem'
goes back to the Baudhayana Sulvasutra, circa, 800 B.C.E.
Various geometric principles were enunciated and constructions (of
fire altars) based on them were described in the Sulvasutras (rules of
mensuration).

Pythagoras' theorem in the Baudhayana Sulvasutra
The diagonal of an oblong produces by itself both the areas which the
two sides of the oblong produce separately (i.e., the square of the
diagonal is equal to the sum of the squares of the two sides).
(Verse 48, Chapter 1)
Introduction of 'zero'.
Decimal place-value system.
A Ninth Century inscription in a temple in Gwalior with the
number 270 appearing in it (as one side of a garden) bears testimony
to the early writing of numbers in a way that is now common place.

Courtesy: Bill Casselman
Aryabhata I (476 550)
Algorithm known as Kuttaka (pulveriser) for solving the
indeterminate equation:
by = ax + c
in integers.
Formulae for sums of progressions involving squares and cubes.
Algorithms for finding square roots and cube roots (these crucially
use the decimal place-value system for representing numbers).
A value of :


100 plus 4, multiplied by 8, and added to 62,000: this is
approximately the measure of the circumference of a circle of
diameter 20,000.
This gives:
,
seen to be accurate to four decimal places.
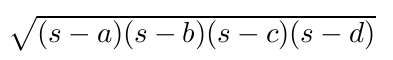
Brahmagupta (598 665)
Well known for his identity for products of sums of squares:
(a2 + b2)(c2 + d2) = (ad
bc)2 + (ac + bd)2.
Formula for areas of cyclic quadrilaterals:
Area =
a, b, c and d being the sides, and s = (a + b + c + d)/2 the semi-
perimeter.
Sridhara (8th Century)
- Formula for the solution of a general quadratic equation in one
variable as we know it today.
Mahavira (9th Century)
- Simultaneous equations with linear and quadratic expressions.
- Empirical rules for computing the area and perimeter of an ellipse.
Jayadeva (9th century) and Bhaskara II (12th century)
- Are well known for their Chakravala (cyclic) method for solving
indeterminate quadratic equations including what came to be known as
'Pell's equation'.
- Several topics in Arithmetic, Algebra, Geometry, Trigono-
metry, etc. saw a high point in the books of Bhaskara II.
Madhava of Sangamagrama (1340 1425)
Definitive progress made towards calculus.
- Invented the Taylor series method.
- Rigorous mathematical analysis and
- Anticipated ('Gregory series') expansion for /4.
Nilakantha Somayaji (1444 1545) of the 'Madhava School' made
considerable progress in mathematical analysis which anticipated the work
of later western analysts, contributing to the rise of calculus.

The Modern Period (20th Century)
The Giants
Srinivasa Ramanujan
1887 - 1919
Harish-Chandra
1923 - 1983
S. Chandrasekhar
1910 - 1995
First Half of the 20th Century
Important mathematical developments took place in the following
institutes:
Calcutta University
University of Madras
Allahabad University
Banaras Hindu University
Aligarh Muslim University
Punjab University
Indian Statistical Institute
Indian Institute of Science
Some important mathematicians
Ananda Rau (1893 1966)
R. Vaidyanthaswamy (1894 1960)
R. C. Bose (1901 1987)
S. S. Pillai (1901 1950)
T. Vijayaraghavan (1902 - 1955)
S. Chowla (1907 1995)
S. Minakshisundaram (1913 1968)
Latter half of the 20th Century
In the post independence era, several new institutions dedicated to
research and teaching were set up. Notable among them are:
Harish Chandra Research Institute, Allahabad
Indian Institutes of Technology (locations spread over the country).
Institute of Mathematical Sciences, Chennai (Madras)
Tata Institute of Fundamental Research, Mumbai (Bombay)
Organizations
Two national societies:
The Indian Mathematical Society
The Ramanujan Mathematical Society
Several regional mathematical societies.
The National Board for Higher Mathematics
Three Academies of Science
The Indian National Science Academy (New Delhi)
The Indian Academy of Sciences (Bangalore)
The National Academy of Sciences, India (Allahabad)
The Department of Science and Technology, Government of India
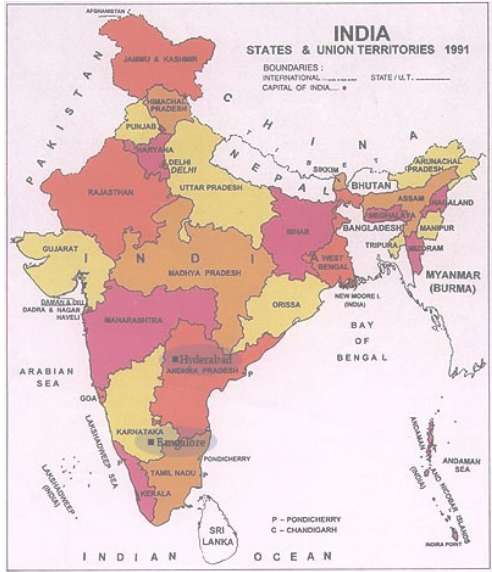
ICM, 2010
Dates: Thursday, August 19 to Friday, August 27, 2010
Venue: Hyderabad International Convention Centre, Hyderabad
Located in the center of India
Historical City
Home to the University of Hyderabad, the Osmania
University and the Jawaharlal Nehru Technological
University
International Airport
State of the art convention centre (proposed venue of ICM 2010).
World class hotel accommodation
General Assembly of the IMU, 2010
Dates: Monday, August 16 and Tuesday, August 17, 2010
Venue: Bangalore
Garden City of India
Largest concentration of institutions devoted to research in science
and engineering.
Seat of the Indian Academy of Sciences
International Airport
Tourism: Hub for Mysore, Belur, Halebidu,
Shravanabelagola, Hampi, ...






Rendez-Vous
Bangalore and Hyderabad
August, 2010
Welcome to India